This is an expanded note prepared for a 40-minute elementary introduction to the Birch and Swinnerton-Dyer conjecture presented at the farewell party for Chen-Yu Chi, who was leaving Harvard after his 8 years as a graduate student and a junior fellow here. As majority of the audience are in fields orthogonal to number-theoretic studies, such a talk can easily succeed in convincing the audience that there is a dry, ridiculous, but famous conjecture coming out of nothing; crazy number theorists have wasted their life to contribute to the list of partial results, which cannot even be claimed to be a long list.
Hopefully I have failed in that way.
Our main sources are [1], [2] and [3].
 Diophantine equations
Diophantine equations
A Diophantine equation is a polynomial equation with integer unknowns, the study of which dates back to the ancient Greeks. The word "Diophantine" comes from the Greek mathematician Diophantus of Alexandria of the 3rd century, the author of a series of books titled Arithmetica. In 1637, Pierre de Fermat scribbled the famous Fermat's last theorem on the margin of his own copy of Arithmetica. The efforts toward its proof has been widely regarded as one of the most glorious stories of mathematics of all time.
Diophantus studied many problems which are essentially quadratic equations from the modern point of view. For example, which integer triples  are the lengths of a right triangle? In other words, what are the rational solutions to the equation
are the lengths of a right triangle? In other words, what are the rational solutions to the equation  ? Notice that the latter equation defines a smooth curve
? Notice that the latter equation defines a smooth curve 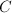 of genus 0 and we are concerned with the set of rational points
of genus 0 and we are concerned with the set of rational points 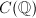 .
.
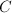 be a smooth projective curve of genus 0 defined over a field
be a smooth projective curve of genus 0 defined over a field  . Then
. Then 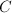 is isomorphic to
is isomorphic to 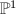 over
over  if and only if
if and only if 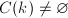 .
.
So the problem of finding all rational points of a genus 0 curve reduces to finding one rational point. Because each genus 0 curve can be embedded into 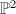 as a plane conic, over a number field, this is achieved by a special case of the Hasse principle for quadratic forms. For example, when
as a plane conic, over a number field, this is achieved by a special case of the Hasse principle for quadratic forms. For example, when 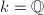 ,
, 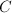 has a point over
has a point over 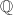 if and only if it has a point over
if and only if it has a point over 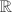 and over every local field
and over every local field 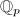 . The latter can be determined handily using Hilbert symbols.
. The latter can be determined handily using Hilbert symbols.
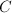 of genus
of genus 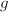 defined over
defined over  , how do we understand
, how do we understand 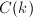 ?
?
The following theorem of Faltings is rather remarkable.
In particular, we know that for any 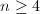 , the Fermat equation
, the Fermat equation 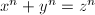 has at most finitely many solutions, which is far beyond the scope of any elementary methods. Faltings' 18-page proof uses a wide range of techniques from algebraic number theory and algebraic geometry.
has at most finitely many solutions, which is far beyond the scope of any elementary methods. Faltings' 18-page proof uses a wide range of techniques from algebraic number theory and algebraic geometry.
Finding these finitely many points is another interesting unsolved problem which we will not go into here. Let us concentrate on the case of genus 1 curves. Let 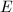 be a smooth curve of genus 1 with a rational point
be a smooth curve of genus 1 with a rational point  (such a curve is called an elliptic curve), then
(such a curve is called an elliptic curve), then  admits a group law with the identity element
admits a group law with the identity element 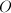 . In particular,
. In particular, 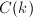 is an abelian group. The old Mordell-Weil theorem asserts that this group cannot be enormous.
is an abelian group. The old Mordell-Weil theorem asserts that this group cannot be enormous.
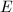 be an elliptic curve over a number field
be an elliptic curve over a number field  , then
, then 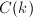 is finitely generated.
is finitely generated.
In other words, as an abstract group, 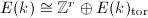 can be decomposed as a direct some of finitely many copies of
can be decomposed as a direct some of finitely many copies of  and a finite abelian group. Here the integer
and a finite abelian group. Here the integer  is called the rank of
is called the rank of 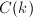 . Notice that
. Notice that 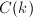 can be both finite (when
can be both finite (when 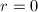 ) and infinite (when
) and infinite (when 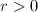 ). This somehow reflects the tension between the infiniteness on curves of genus 0 and finiteness on curves of higher genus, making the case of elliptic curves most interesting and intricate.
). This somehow reflects the tension between the infiniteness on curves of genus 0 and finiteness on curves of higher genus, making the case of elliptic curves most interesting and intricate.
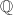 can be realized as a plane cubic using the Weierstrass equation
can be realized as a plane cubic using the Weierstrass equation 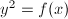 , where
, where ![$f(x)\in \mathbb{Q}[x]$](./latex/latex2png-BSD_246174086_-5.gif) is a cubic polynomial, with the point
is a cubic polynomial, with the point 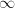 serving as the identity element
serving as the identity element 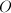 here. The group law can be realized as the secant-tangent process. For example, let
here. The group law can be realized as the secant-tangent process. For example, let 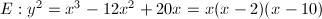 . We easily find the rational points
. We easily find the rational points 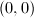 ,
, 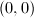 ,
,  and
and 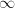 . These four are torsion points, all having order 2. The solution
. These four are torsion points, all having order 2. The solution 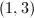 can also be inspected and it generates infinitely many solutions (e.g., twice of it is
can also be inspected and it generates infinitely many solutions (e.g., twice of it is 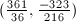 , which is hard to observe directly). As an abstract group,
, which is hard to observe directly). As an abstract group, 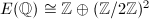 .
.
The arithmetics of elliptic curves is also a significant node of the giant web of arithmetic problems. Some examples are in order.
 is equal to
is equal to 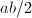 for some positive rational triple
for some positive rational triple 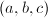 satisfying
satisfying 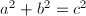 . A bit of elementary calculation shows that the elliptic curve
. A bit of elementary calculation shows that the elliptic curve 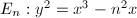 has a rational point
has a rational point  . Moreover, it can be shown that
. Moreover, it can be shown that 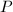 must be of infinite order. Conversely, such a point of infinite order gives us back a rational triple
must be of infinite order. Conversely, such a point of infinite order gives us back a rational triple 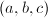 . Thus showing that
. Thus showing that  is a congruent number is equivalent to showing that the elliptic curve
is a congruent number is equivalent to showing that the elliptic curve  has positive rank.
has positive rank.
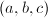 is a nontrivial solution to
is a nontrivial solution to 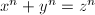 , then the elliptic curve
, then the elliptic curve 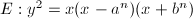 is not modular (this is proved by Ribet (1990) by proving the epsilon conjecture). Wiles (1995) showed that all semistable elliptic curves over
is not modular (this is proved by Ribet (1990) by proving the epsilon conjecture). Wiles (1995) showed that all semistable elliptic curves over  (e.g., Frey's curve) are modular, thereby finishing the proof of Fermat's last theorem. In 2001, Breuil-Conrad-Diamond-Taylor extended the modularity to all elliptic curves over
(e.g., Frey's curve) are modular, thereby finishing the proof of Fermat's last theorem. In 2001, Breuil-Conrad-Diamond-Taylor extended the modularity to all elliptic curves over  .
.
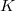 be a number field. Poonen (2002) proved that if there is an elliptic curve
be a number field. Poonen (2002) proved that if there is an elliptic curve 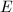 over
over 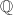 such that
such that 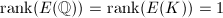 , then Hilbert's 10th problem has a negative answer over the ring of integers
, then Hilbert's 10th problem has a negative answer over the ring of integers 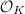 : there does not exist an algorithm to decide whether a Diophantine equation with
: there does not exist an algorithm to decide whether a Diophantine equation with 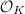 -coefficients has an
-coefficients has an 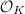 -solution or not.
-solution or not.
 The Birch and Swinnerton-Dyer conjecture
The Birch and Swinnerton-Dyer conjecture
The following statements and conjectures apply for any elliptic curves 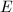 over a global field
over a global field  . For simplicity, we consider the case
. For simplicity, we consider the case 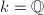 .
.
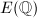 ?
?
Mazur's torsion theorem classifies all possible torsion part of 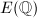 . His proof is a paradigm of using scheme-theoretic methods to draw concrete arithmetic consequences.
. His proof is a paradigm of using scheme-theoretic methods to draw concrete arithmetic consequences.
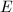 be an elliptic curve over
be an elliptic curve over 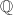 , then
, then 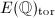 is one of the following groups:
is one of the following groups: 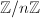 for
for  and
and 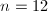 or
or 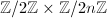 for
for  .
.
On the contrary, we understand far less about the rank  of
of 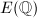 . For example, it is not known which integer values
. For example, it is not known which integer values  can take; it is even not known whether
can take; it is even not known whether  can take arbitrarily large values. People intend to believe the following conjectures about the rank
can take arbitrarily large values. People intend to believe the following conjectures about the rank  .
.
Tate-Shafarevich (1967) proved the unboundedness of the rank over functions fields. The unboundedness in the number field case is still unknown: the current record over 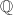 is kept by Elkies (2006), he found an elliptic curve with at least 28 independent
is kept by Elkies (2006), he found an elliptic curve with at least 28 independent 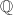 -points via the theory of Mordell-Weil lattices of elliptic K3 surfaces and searching techniques.
-points via the theory of Mordell-Weil lattices of elliptic K3 surfaces and searching techniques.
It is not even known whether the average rank has an upper bound until the recent seminal work of Bhargava-Shankar (2010). They used methods from the geometry of numbers to count certain integral orbits of the space of binary quartic forms to show that the average rank, if it exists, is less than 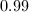 .
.
Miraculously, the rank of an elliptic curve, which we do not have understand well, is related to the analytic properties of its 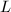 -function
-function 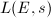 . To motivate this, recall that associated to each algebraic variety
. To motivate this, recall that associated to each algebraic variety 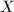 over a finite field
over a finite field 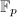 , its zeta function
, its zeta function 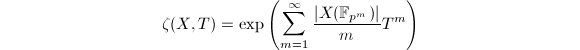 is a rational function of
is a rational function of  (the Weil conjecture). In our case, taking the reduction mod
(the Weil conjecture). In our case, taking the reduction mod 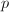 of an elliptic curve
of an elliptic curve 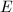 over
over 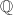 gives an elliptic curve
gives an elliptic curve 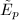 over
over 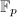 (here we ignore what happens for the bad primes). Its zeta function is
(here we ignore what happens for the bad primes). Its zeta function is 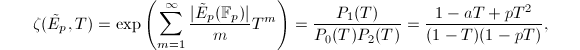 where
where 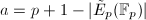 . Analogous to the Euler factors of the Riemann zeta function, we define the local
. Analogous to the Euler factors of the Riemann zeta function, we define the local 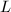 -factor of
-factor of 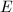 to be
to be  When evaluating its value at
When evaluating its value at 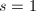 , we retrieve the arithmetic information at
, we retrieve the arithmetic information at 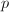 ,
, 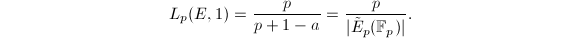 Notice that each point in
Notice that each point in 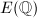 reduces to a point in
reduces to a point in 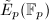 . So when
. So when 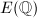 has high rank, then
has high rank, then  tends to be small. Birch and Swinnerton-Dyer did numerical experiments and suggested the heuristic
tends to be small. Birch and Swinnerton-Dyer did numerical experiments and suggested the heuristic 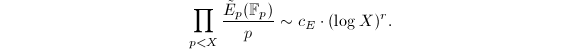
The 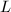 -function of
-function of 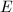 is defined to be the product of all local
is defined to be the product of all local 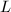 -factors,
-factors, 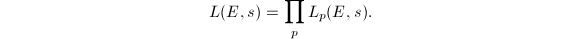 Formally evaluating the value at
Formally evaluating the value at 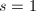 gives
gives 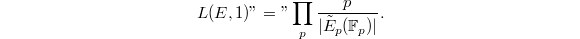 So intuitively the rank of
So intuitively the rank of 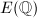 will correspond to the value of
will correspond to the value of 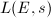 at 1: the larger
at 1: the larger  is, the "smaller"
is, the "smaller" 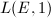 is. However, the value of
is. However, the value of 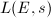 at
at 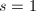 does not make sense since the product of
does not make sense since the product of 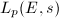 only converges when
only converges when 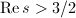 . Nevertheless, if
. Nevertheless, if 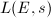 can be continued to an analytic function on the whole of
can be continued to an analytic function on the whole of 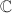 , it may be reasonable to believe that the behavior of
, it may be reasonable to believe that the behavior of 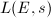 at
at 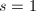 contains the arithmetic information of the rank of
contains the arithmetic information of the rank of 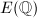 . The famous Birch and Swinnerton-Dyer conjecture asserts that
. The famous Birch and Swinnerton-Dyer conjecture asserts that
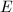 be an elliptic curve over a global field
be an elliptic curve over a global field  , then the order of vanishing of
, then the order of vanishing of 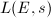 at
at 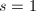 is equal to the rank of
is equal to the rank of 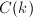 .
.
For this reason, we call the number 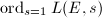 the analytic rank of
the analytic rank of 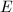 . Even explicit constants are conjecturally described:
. Even explicit constants are conjecturally described: 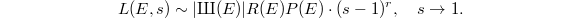 Here
Here 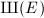 is the Tate-Shafarevich group, measuring the failure of the Hasse principle for
is the Tate-Shafarevich group, measuring the failure of the Hasse principle for 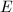 -torsors;
-torsors; 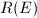 is the regulator with respect to the Neron-Tate height pairing on
is the regulator with respect to the Neron-Tate height pairing on 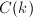 ;
;  is the period, a product of local periods obtained by integrating the Neron differential on
is the period, a product of local periods obtained by integrating the Neron differential on 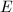 .
.
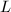 -functions, the BSD conjecture can be true only if the product
-functions, the BSD conjecture can be true only if the product 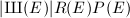 is isogeny-invariant. This is proved by Cassels (1965) assuming the finiteness of
is isogeny-invariant. This is proved by Cassels (1965) assuming the finiteness of 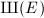 , even though each individual factor may change under isogeny!
, even though each individual factor may change under isogeny!
This remarkable conjecture has been chosen as one of the seven Millennium Prize problems by the Clay Institute, with a million-dollar prize for its solution. It is even more remarkable when we notice that it is so "meaningless": the left-hand-side has no meaning because we did not even know whether 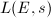 was defined round
was defined round 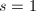 at the time when conjecture was formulated (except for elliptic curves with complex multiplication due to the work of Deuring and Weil in the 1950s), and the right-hand-side has no meaning either because we do not know in general that
at the time when conjecture was formulated (except for elliptic curves with complex multiplication due to the work of Deuring and Weil in the 1950s), and the right-hand-side has no meaning either because we do not know in general that 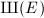 is finite! Nevertheless, this is indeed a good situation for mathematicians: we have more flexibility to figure out what the truth is.
is finite! Nevertheless, this is indeed a good situation for mathematicians: we have more flexibility to figure out what the truth is.
Here are some consequences of the BSD conjecture.
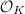 for any number field
for any number field 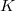 .
.
 Progress to date
Progress to date
As we mentioned, the formulation of the BSD conjecture relies on the following two conjectures.
Thanks to the modularity theorem of Wiles and others, we now know 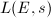 can be always continued analytically to the whole of
can be always continued analytically to the whole of 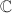 , but the finiteness of
, but the finiteness of  is still largely open: in each case that the finiteness of
is still largely open: in each case that the finiteness of  is known, the BSD conjecture of
is known, the BSD conjecture of 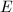 is proved along with it too.
is proved along with it too.
There is much evidence in favor of the BSD conjecture, we now list a few of them. The function field case is of better shape than the number field case due to
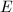 over a function field
over a function field  if and only if
if and only if 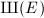 is finite.
is finite.
The best general result to date for the BSD conjecture over number fields is due to the groundbreaking Gross-Zagier formula relating the central derivative 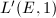 and the heights of Heegner points on
and the heights of Heegner points on 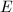 defined over an imaginary quadratic field. The Gross-Zagier formula has the following implication.
defined over an imaginary quadratic field. The Gross-Zagier formula has the following implication.
Building on the Gross-Zagier formula and his theory of Euler systems, Kolyvagin proved that
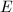 over
over 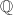 with
with 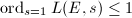 . In particular,
. In particular, 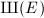 is finite for all such elliptic curves.
is finite for all such elliptic curves.
There is a weaker version of BSD concerning the parity of ranks.
Notice that the parity of the analytic rank is directly related to the root number, the sign of the functional equation of 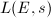 . The parity conjecture is more tractable because the root number can be defined independent on any conjectures using local Galois actions on the Tate module.
. The parity conjecture is more tractable because the root number can be defined independent on any conjectures using local Galois actions on the Tate module.
Nekovar (2006) extended the parity conjecture to totally real fields. Dokchitser-Dokchitser (2009) proved the 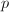 -parity conjecture, which allows us to weaker the condition of finiteness of
-parity conjecture, which allows us to weaker the condition of finiteness of 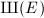 to the finiteness of the
to the finiteness of the 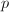 -primary part
-primary part ![$\Sha(E)[p^\infty]$](./latex/latex2png-BSD_217110488_-5.gif) for a prime
for a prime 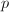 .
.
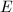 over
over 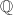 with
with 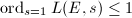 and conductor
and conductor 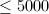 by Stein and others. There is no elliptic curve
by Stein and others. There is no elliptic curve 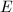 over
over 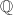 with analytic rank
with analytic rank  for which
for which 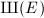 can be shown to be finite, nevertheless, there is numerical evidence (up to a rational factor) for many cases with higher analytic rank.
can be shown to be finite, nevertheless, there is numerical evidence (up to a rational factor) for many cases with higher analytic rank.
 (when
(when  is large) is still by far a demanding computational problem: there are useful algorithms in practice, but none of them can always guarantee the correct output. The main difficulty again lies in computing the mysterious group
is large) is still by far a demanding computational problem: there are useful algorithms in practice, but none of them can always guarantee the correct output. The main difficulty again lies in computing the mysterious group 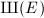 . Assuming the BSD conjecture, we can instead compute the analytic rank. However, because we do not have a Gross-Zagier formula for higher derivatives of
. Assuming the BSD conjecture, we can instead compute the analytic rank. However, because we do not have a Gross-Zagier formula for higher derivatives of 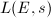 , the analytic rank is hard to compute provably correctly (is
, the analytic rank is hard to compute provably correctly (is  zero or a tiny rational number?). It is still an open problem to find an elliptic curve over
zero or a tiny rational number?). It is still an open problem to find an elliptic curve over 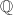 of provably correct analytic rank
of provably correct analytic rank 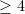 .
.
References
[1]Lectures on the conjecture of Birch and Swinnerton-Dyer, Arithmetic of L-functions, AMS PCMI publications, 2011, 169-210.
[2]Ranks "cheat Sheet", 2011, http://math.uci.edu/~asilverb/connectionstalk.pdf.
[3]The Arithmetic of Elliptic Curves (Graduate Texts in Mathematics), Springer, 2010.
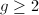 over a number field
over a number field 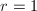 : 50%;
: 50%; 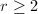 : 0%. The average rank is
: 0%. The average rank is 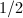 .
.
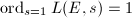 , then
, then 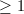 .
.
 .
.